A couple of months ago, I read Paul J. Steinhardt's wonderful book The Second Kind of Impossible, about his (and others') search for quasicrystals -- a bizarre form of matter that is crystalline but aperiodic (meaning it fills the entire space in a regular fashion, but doesn't have translational symmetry). Here's an artificial quasicrystal made of aluminum, palladium, and manganese:
As the above photograph shows, they can be created in the lab, but Steinhardt believed they could occur naturally -- and he finally proved it, in a meteorite sample he and his team found in a remote region of Siberia.
I was immediately reminded of Steinhardt's aperiodic crystals when I read a paper in Chaos: An Interdisciplinary Journal of Nonlinear Science, by Francesca Bertacchini, Pietro Pantano, and Eleanora Bilotta, of the University of Calabria, who were experimenting with another nonrandom but chaotic shape -- a "strange attractor."
A strange attractor is a concept from fractals and chaos theory, and represents a value toward which a perturbed system tends to evolve. Chaos theory has been around for a while, but came to most people's attention from Jurassic Park, when the character Ian Malcolm (portrayed in memorable fashion by Jeff Goldblum) is explaining the unpredictability of complex systems using the direction a drop of water rolls on a relatively (but not perfectly) flat surface, in this case, the back of someone's hand. Systems like that one tend to rush far out of equilibrium -- once the drop starts to move, it keeps going -- but some systems settle into a set of loops or spirals, as if something in the middle was drawing them in.
Thus the name strange attractor.
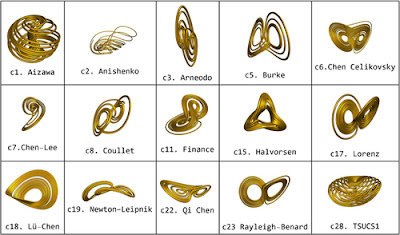
These systems, when mapped out, create some beautiful patterns -- like Steinhardt's quasicrystals, with the superficial appearance of regularity, but without any repeats or obvious symmetries. Bertacchini et al. used the mathematical functions describing the system to drive a 3-D printer and actually create models of what strange attractors look like. The team was struck with how beautiful the shapes were, and had a goldsmith fashion them as jewelry. Here are a few of their creations:
The authors write:
[We used] a chaotic design approach used to develop jewels from chaotic design. After presenting some of the most important physical systems that generate chaotic attractors, we introduced the basic steps of this approach. This approach exploits a number of fundamental characteristics of chaotic systems. In particular, the parametric design approach exploits the concept of extreme sensitivity to the initial data that leads to evolutionary transformations of dynamic systems, not only along the traditional routes to chaos and through qualitative changes in the starting chaotic system, but also through changes in the basic parameters of the system, which create infinite chaotic forms. Such phase spaces, therefore, represent an enormous potential to be exploited in the design of artistic objects, whether they are jewelry pieces or other objects of abstract art. In the computational approach used, each shape is unique and it is identified by a set of parameters that almost constitute its precise value. This leads to the creation of unique artistic forms and, thus, to the customization of products in the case of jewelry pieces, which exploits chaotic design as a methodology.
The whole thing brings up for me the mysterious question of what we find beautiful -- and how so often, it's a balance between predictability and unpredictability, between symmetry and randomness. It reminds me of the quote from the brilliant electronic music pioneer Wendy Carlos: "What is full of redundancy is predictable and boring. What is free from all structure is random and boring. In between lies art."


No comments:
Post a Comment